He mentioned such 'mixed' measures several times, see e.g. the depression CES-D one:
"items appear to be a mixture of effect and causal indicators. For instance, "I felt depressed" and "I felt sad" appear to be effect indicators of depressed mood. That is, we expect that a change in the latent depression variable leads to a change in responses to these items. However, "I felt lonely" could be a causal indicator of the same construct in that loneliness may cause depression rather than vice versa. Alternatively, loneliness could be a separate dimension that requires several indicators to measure it. To further complicate things, one could argue that some items are reciprocally related to depression.
For example, individuals may become depressed because they think people dislike them, which makes them appear unattractive, thus other people may avoid them and actually dislike them (i.e., the low affect may be unattractive and offputting)." [1:311]
Note 1:
Les Hayduk explored the 'reciprocally related' ('reactive') indicators in detail in [5]:
Note 2:
Les also advanced the 'finite cycling' feedback/nonrecursive models in [6], which simply says that infinite cycling is not realistic, so one can conceive of: 1. 1 full cycle; 2. 1 & 1/2 cycle; 3. 2 full cycles; etc. (although more than 2 cycles around the effects add up very little). See also in this [6] great language on why/how one splits an effect η1 -> η2 into 2 such effects, see below the quote, referring to his Fig. 1 below:
“To
identify the estimates of the effects in a model like Figure 1 (in the absence
of additional equality, proportionality, or other constraints on β21
and β12) a variable like ξ1 is required (Rigdon, 1995).
The direct effect of ξ1 on η1, and the important absence
of a direct effect of ξ1 on η2, implies that the
covariance between ξ1 on η2 arises from the basic
indirect effect of ξ1 on η2 (namely γ11
β21),
which is enhanced by the loop L = β21 β12.
The relegation of β12 to a purely enhancing role in accounting for
the covariance between ξ1 and η2 differentiates or disentangles
β21 from β12 and makes it possible to obtain separate
estimates of these effects. In the Figure 1 model, ξ2 similarly
contributes to disentangling the reciprocal effects, so as long as the γ11
and γ22 effects are substantial, the β21 and β12
effects in this model should be overidentified (given the independence of the
disturbance terms).” [6: 660-661].
1. Bollen, K. A., & Lennox, R. (1991). Conventional wisdom on measurement: A structural equation perspective. Psychological Bulletin, 110(2), 305-314. doi:10.1037/0033-2909.110.2.305
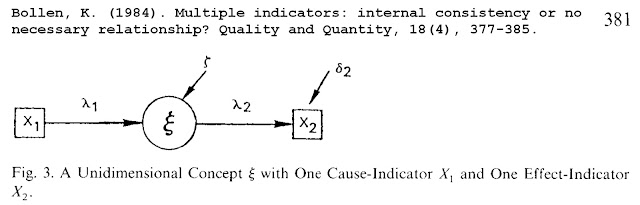
2. Bollen, K. (1984). Multiple indicators: internal consistency or no necessary relationship? Quality and Quantity, 18(4), 377-385.
3. Bollen, K. A., Glanville, J. L., & Stecklov, G. (2001). Socioeconomic status and class in studies of fertility and health in developing countries. Annual Review of Sociology, 153-185.
4. Bollen, K. A., & Bauldry, S. (2011). Three Cs in measurement models: Causal indicators, composite indicators, and covariates. Psychological Methods, 16(3), 265-284. doi:10.1037/a0024448
5. Hayduk, L. A., Robinson, H. P., Cummings, G. G., Boadu, K., Verbeek, E. L., & Perks, T. A. (2007). The weird world, and equally weird measurement models: Reactive indicators and the validity revolution. Structural Equation Modeling: A Multidisciplinary Journal, 14(2), 280-310.
6. Hayduk, L. A. (2009). Finite Feedback Cycling in Structural Equation Models. Structural Equation Modeling: A Multidisciplinary Journal, 16(4), 658-675.